Convolution Theorem Laplace Transform Examples

Lets assume that you have a mixer or a smoothie maker. You put some fruits in it.

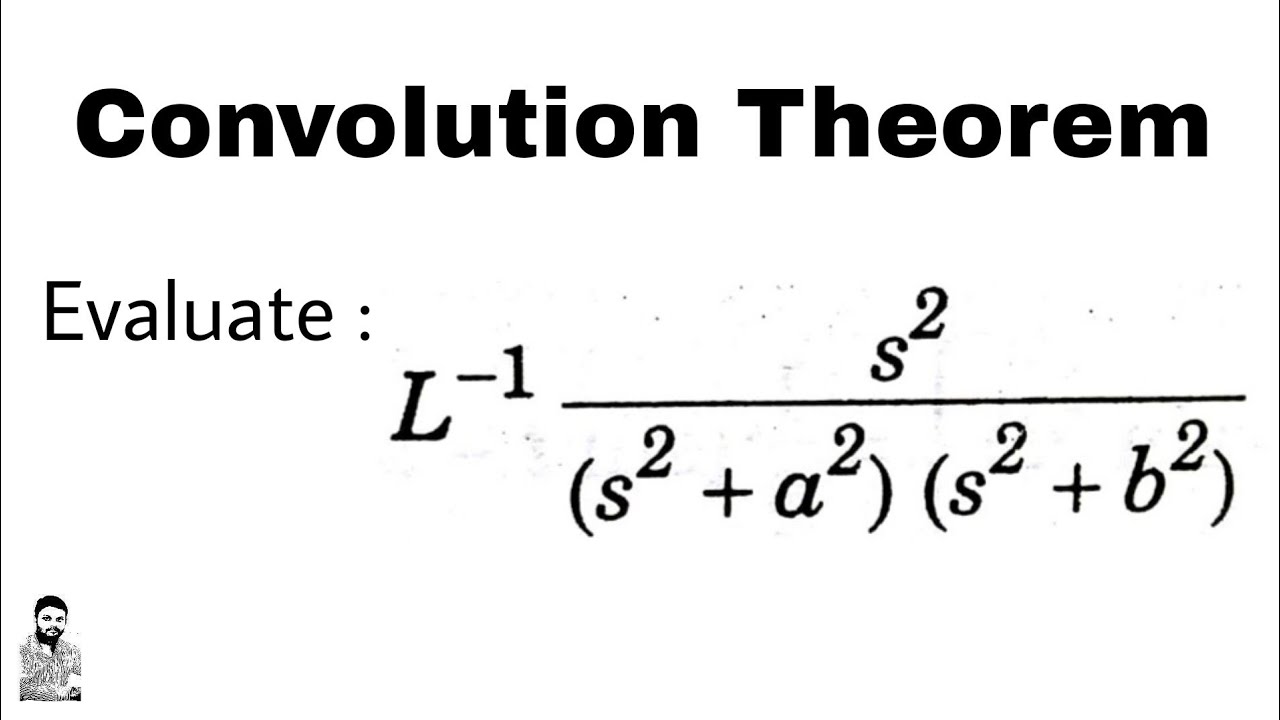
20 Convolution Theorem Problem 2 Inverse Laplace Transforms Youtube

A power series may converge for some values of x but diverge for other.

. A series of free Differential Equations Lessons. L t 0 f1τf2tτ dτ 0 f1τ τ e. As we know that the Laplace transform of sin at a s2 a2.

Example 2 Solve the following IVP 4y y gt y0 3 y0 7 4 y y g t y 0 3 y 0 7. More generally convolution in one domain eg time domain equals point-wise multiplication in the other domain eg frequency domain. The convolution theorem for Laplace transform states that.

Lets say you would put two kinds of fruits ie. L f g L f L g. Where the right hand side is a double integral over the angular region bounded by the lines τ 0 τ 0 and τ t τ t in the first quadrant of the tτ t τ -plane.

Try the free Mathway calculator and problem solver below to practice various math topics. The meaning of other integral depends on types of functions of interest. Each fruit has a different weight banana1 50g banana2100g banana378g Apple160g etc.

The convolution theorem can be used to provide a formula for the solution of an initial value problem for a linear constant coefficient differential equation in which the forcing function is complicated to determine its Laplace transform. Similarly the set of values for which Fs converges conditionally or absolutely is known as the region of conditional convergence or simply the region of convergence ROC. The standard proof uses Fubini-like argument of switching the order of integration.

Statement The convolution of two signals in time domain is equivalent to the multiplication of their spectra in frequency domain. Example 1 Use a convolution integral to find the inverse transform of the following transform. This is a consequence of Fubinis theorem and Moreras theorem.

In mathematics the convolution theorem states that under suitable conditions the Fourier transform of a convolution of two functions or signals is the pointwise product of their Fourier transforms. Find the Laplace transform of function f t defined by f t 1 Solution to Example 1 Use the definition of the Laplace transform given above F s 0 f t e s t d t f t 1 Over the interval of integration 0 hence F s simplifies to F s 0 e s t d t Calculate the above improper integral as follows. The Laplace transform of f t sin t is L sin t 1 s2 1.

First lets take a look at fourier transform. Changing the of integration we write. You would put three bananas and four apples.

Convolution has the following properties which work as you would expect. Try it on your own before looking at. Example 851 Compute if f t et and.

The Laplace transform deflnitionexamples propertiesformulas linearity theinverseLaplacetransform timescaling exponentialscaling timedelay derivative integral multiplicationbyt. The convolution theorem will also prove useful in connection with the use of the Laplace transform for the solution of differential equations a topic we pursue in the next section of this text. Constant Property c is a constant Commutative Property Associative Property Distributive Property Before we go on lets do an example.

Example 13612 A Laplace Transform Inversion. Using The Convolution Theorem To Solve An Initial Value Problem. Get complete concept after watching this videoTopics covered under playlist of Laplace Transform.

The Fourier transform of a continuous-time function 𝑥𝑡 can be defined as mathrmXomegaint_-inftyinftyxte-jomega tdt Convolution Property of Fourier Transform. 0 d τ τ e s t f t τ g τ d t 0 d t 0 t e s t f t τ g. It transforms variables from time-domain to.

Convolution theconvolutionofsignalsfandgdenotedh fgisthesignal ht Zt 0. Further suppose that and. The Laplace transform is analytic in the region of absolute convergence.

Lets use the convolution theorem to. Then 838 LaplaceTransform f t t s LaplaceTransform g t t s Note that is called the convolution integral. The Laplace transform of the convolution integral.

Theorem 32 Convolution Theorem Suppose that f t and g t are piecewise continuous on and both of exponential order b. The Laplace Transform is an operator that maps the input to the output of a linear differential equation involving derivatives of functions. Intro to Power Series A power series is a series of the form X1 n0 a nx x 0n a 0 a 1x x 0 a 2x x 02 It can be thought of as an in nite polynomial The number x 0 is called the center.

H s 1 s2 a22 H s 1 s 2 a 2 2 Show Solution Convolution integrals are very useful in the following kinds of problems. Definition Transform of Elementary Functions Properties o. Try the given examples or type in your own problem and check your answer with the step-by-step.

According to the definition of Laplace transform one has. In laplace transforms can be solved using laplace transform of convolution theorem is relatively simple examples of. Ad Browse Discover Thousands of Science Book Titles for Less.

The Convolution And The Laplace Transform.

Convolution Theorem Laplace Transforms Example Problem 1 Youtube

Csir Ugc Net Convolution Theorem In Laplace Transform Part 1 Offered By Unacademy

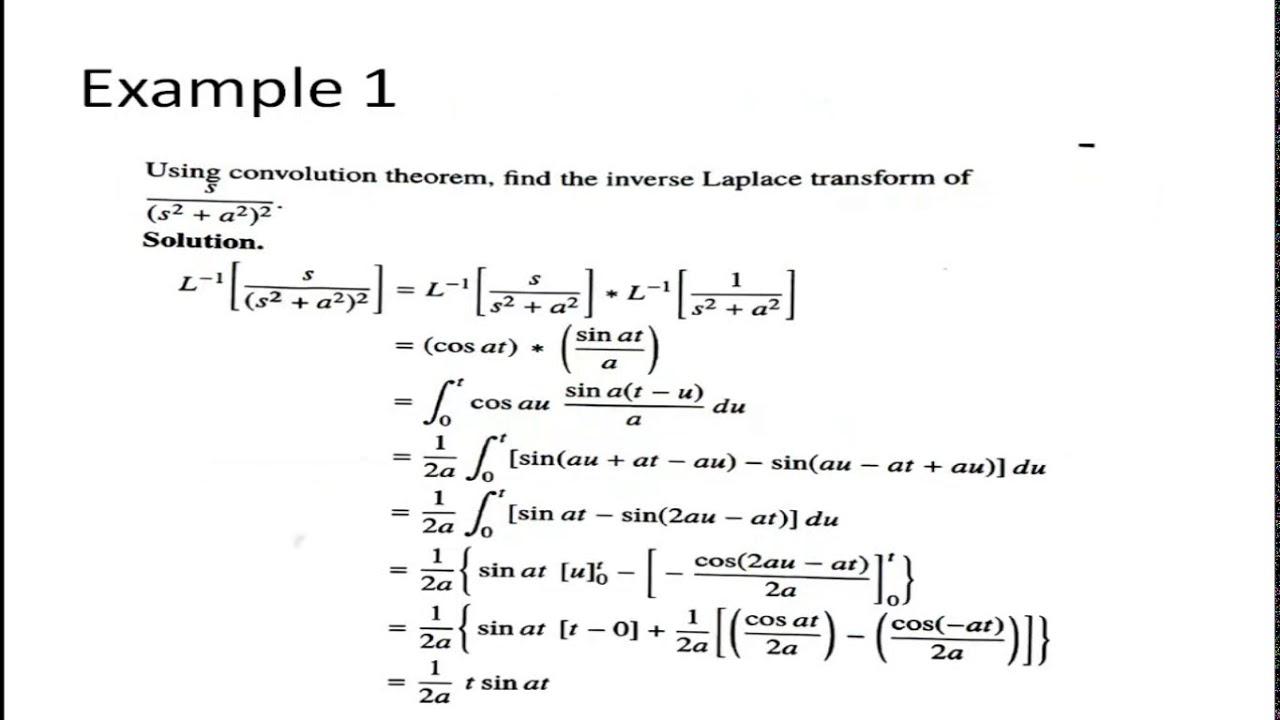
B Tech S2 S4 Laplace Transforms Convolution Theorem Youtube

Using The Convolution Theorem To Find The Inverse Laplace Transform Youtube